The Full Width at Half Maximum, or "FWHM", is an important
measure for quantifying
image sharpness. This feature is available in Mira Pro x64 and all Mira Pro
platforms. One application of FWHM is for determining apertures size for aperture photometry. FWHM is
also important for characterizing the size of the point spread
function as a measure of focus, atmospheric turbulence, or
optical performance. Although FWHM is a mathematically well-defined
quantity, it is difficult to measure accurately in real data. FWHM
is measured from the image of a point source such as a star or a specular
highlight reflection. A common shortfall of imaging software is to produce
accurate estimates of the FWHM, especially noticeable in the
variations reported for bright and
faint sources. The technique Mira uses involves fitting a Gaussian +
Constant model to data around the source. This method leads to
scientifically accurate
results, independent of brightness as they should be. Mira provides
two ways to estimate the FWHM: You can directly measure and tabulate
FWHM and other parameters by clicking on a point source, or you can
measure it indirectly using a Radial Profile plot. The Radial
Profile technique shows data from all azimuths around the point
source. This method gives visual verification that the FWHM value is
correct for the data.
The pictures below compare the FWHM measured using a Radial Profile plot for stars of different brightness. Since all stars were taken from a small region of the image, the point spread functions should be similar except for scaling in brightness. Therefore, the FWHM values should all be the same, within random variation due to noise. In this experiment, we used objects covering a range of 13,200 ADU above background down to 11 ADU above background (3 sigma's). Does your current software produce results this accurate and consistent?
FWHM = 6.484
Peak 13,200
FWHM = 6.503
Peak = 668
The FWHM is virtually identical with the value measured for a star 20 times brighter.
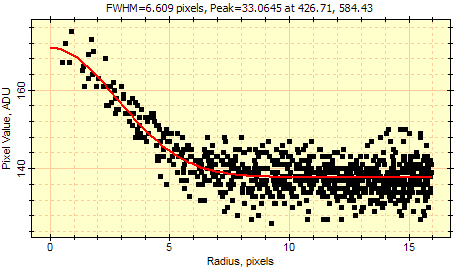
FWHM = 6.609
Peak = 33
The FWHM of this faint star is consistent with the values measured for much brighter objects.
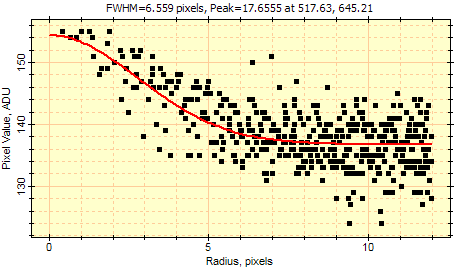
FWHM = 6.559
Peak = 17.6
This star has a peak 4.8 sigma's above the sky. Still, the FWHM estimate is consistent with that of much brighter objects. Pixel values quantized at the 1 ADU level are evident in the sky pixels of this 16 bit integer image.
FWHM = 5.59
Peak = 11.2
This measurement is really down in the noise! The peak central brightness of this star is only 3 sigma's above the sky. This result is a testament to the robustness of both the FWHM and centroid algorithms used by Mira.
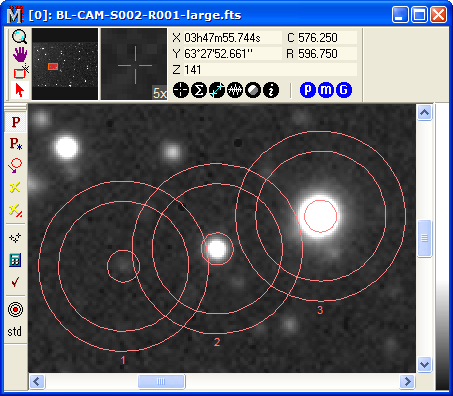
The Source Image
An image window showing the 3 brightest stars being measured using Mira's aperture photometry tool. The faintest star circled peaks at only 33 ADU (9 sigma's) above background. The stars at 4.8 and 3.0 sigma's are almost undetectable in this view.